Previous: The Mathematics of Orbifold Pinball
Up: The Mathematics of Orbifold Pinball
Next: Conway's Notation and the Euler Number

What is an Orbifold?

Definition.

An orbifold is a Hausdorff space which is locally homeomorphic at each point to , where is some finite group, perhaps different for different . The set of s.t. (in other words, points no neighborhood of which is homeomorphic to ) is called the singular locus of the orbifold .

Definition.

An orbifold is called good if it has a covering which is a manifold.

The following amazing theorem is true for 2-orbifolds:

Theorem.

The singular locus of a 2-orbifold has only three possible types of local model:

1. A mirror , where acts by reflections along the line.
2. A cone point of order acting by rotations of order .
3. A corner reflection of order generated by all reflections in two lines which meet at an angle .

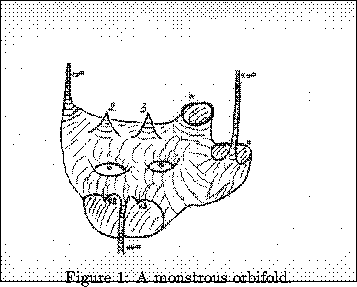
Here is an example which shows some of the features a 2-orbifold may have: