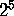Unicursal curves and knot diagrams

A unicursal curve in the plane is a curve that you get when you put down your pencil, and draw until you get back to the starting point. As you draw, your pencil mark can intersect itself, but you're not supposed to have any triple intersections. You could say that you pencil is allowed to pass over an point of the plane at most twice. This property of not having any triple intersections is generic: If you scribble the curve with your eyes closed (and somehow magically manage to make the curve finish off exactly where it began), the curve won't have any triple intersections.

A unicursal curve differs from the curves shown in knot diagrams in that there is no sense of the curve's crossing over or under itself at an intersection. You can convert a unicursal curve into a knot diagram by indicating (probably with the aid of an eraser), which strand crosses over and which strand crosses under at each of the intersections.

A unicursal curve with 5 intersections can be converted into a knot diagram in 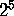ways, because each intersection can be converted into a crossing in two ways. These 32 diagrams will not represent 32 different knots, however.

Assignment

1. Draw the 32 knot diagrams that arise from the unicursal curve underlying the diagram of knot 5-2 shown in the previous section, and identify the knots that these diagrams represent.
2. Show that any unicursal curve can be converted into a diagram of the unknot.
3. Show that any unicursal curve can be converted into the diagram of an alternating knot in precisely two ways. These two diagrams may or may knot represent different knots. Give an example where the two knots are the same, and another where the two knots are different.
4. Show that any unicursal curve gives a map of the plane whose regions can be colored black and white in such a way that adjacent regions have different colors. In how many ways can this coloring be done? Give examples.