Maxima, Minima, and Saddles:

Maxima

Points where the surface is locally below the height of the critical point. A component of the level set disappears as the height passes a maximum. A maximum typically looks like the origin in z = -x^2 - y^2.

Minima

Points where the surface is locally above the height of the critical point. A new component of the level set is formed as the height passes a minimum. A minimum typically looks like the origin in z = x^2 + y^2.

Saddles

Points where every neighborhood of the critical point intersects the plane perpendicular to the height direction. The number of components in the level set usually changes as the height passes a saddle point. If the surface is non-orientable, however, the number of components may stay the same, as is the case with the projective plane drawn by Kuiper with only three critical points.

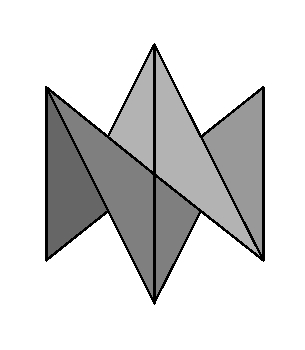
A saddle typically looks like the origin in z = x^2 - y^2. This is a surface that goes up in two places and down in two places. There are more complicated saddles (with more ups and downs). In the case of smooth surfaces, these are not generic: a slight change of direction will break the saddle into two ore more standard saddles. This is not true for polyhedral surfaces, where complex saddles are stable under slight changes of direction. There are also "exotic" saddles that have no smooth counterpart, as in the diagram below.