To see the changing three-dimensional shadows created as this object completes a rotation in four-dimensional space, select any point in the image below to download an animation (486K).
The flat torus is constructed by a very simple formula, namely
[u,v] -> [cos(u + v), sin(u + v), cos(u - v), sin(u - v)]/sqrt(2)where u and v both run from zero to 2 pi. The sum of the squares of these four coordinates is 1 so the object is completely contained in the hypersphere of radius 1 centered at the origin in four-space.
This torus is covered with circles, and when we project stereographically from the pole [0, 0, 0, 1] on the three-sphere, the image in three-space is a torus of revolution covered with circles each of which goes around the torus once in each direction. Any two of these circles are linked in the sense that a disc bounded by one of them is cut exactly once by any other of them.
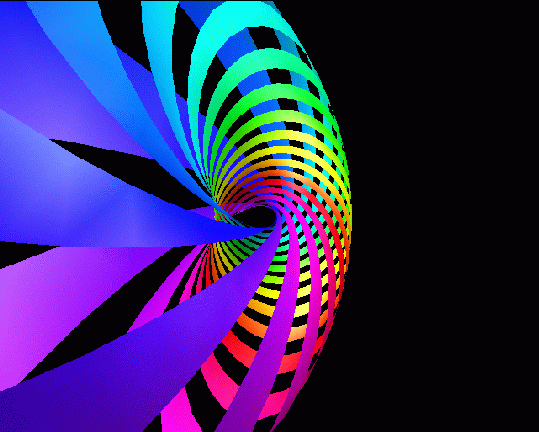
We can get a better idea of the structure of this surface as an object in the three-sphere by rotating the sphere and observing the images down below in our three-dimensional space under stereographic projection. The surface appears to "turn itself inside out" under this transformation. We can see a more symmetrically situated view of this by selecting the picture with the mouse button to show a short animation (274K) of the rotating object.
Half way through the rotation in four-dimensional space, the torus actually passes through the point of projection so the image in three-space is infinitely large, containing straight lines that are the images of circles going through the projection point. By selecting this picture, it is possible to see an animation (386K) of this infinite surface rotating in three-dimensional space.
These surfaces are known as Cyclides of Dupin. All of the bounded ones are of fourth order, meeting any line not tangent to the surface in at most four points. The infinite ones are of third order.
For a non-technical description of this surface, see the author's Scientific American Library volume Beyond the Third Dimension and for a technical article featuring Cyclides of Dupin, see The Spherical Two-Piece Property and Tight Surfaces in Spheres.
For more information on the geometry of the Hopf mapping connected with these circles on the flat torus, see the author's papers Geometry of the Hopf Mapping and Pinkall's Tori of Given Conformal Type, Topology and Mechanics with Computer Graphics: Linear Hamiltonian Systems in Four Dimensions and Torus Decompositions of Regular Polytopes in 4-Space.