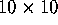 grid to approximate the integral
grid to approximate the integral
In a previous question we used rectangles on a 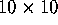 grid to approximate the integral
grid to approximate the integral

where D is the ellipse with axes 6/5 and 1. This is equivalent to the double iterated integral

where 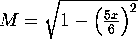 .
.
According to the theory of Riemann sums, this integral should equal the limit of the area of our approximation as the grid gets finer and finer.
How confident are you in your answer? (You may want to compute approximations for more grid sizes.)