Centers of Mass and Centroids

Two Point-Masses

Suppose we have two particles of mass m1 and m2 at positions x1 and x2 , and suppose that the particles are connected by a lightweight inflexible rod. We say the particles form a system or a rigid body. Analyzing the properties of such a system is the first step to understanding the properties of more complicated objects.

One of the most important properties of the system is the center of mass . Intuitively, the center of mass is the location where an object could balance perfectly level on the tip of a pin.

Figure 1: An asymmetric dumbell-shaped object.

Group Discussion

Describe the center of mass for the two particle system if

m1 = m2
m1 > m2
m1 = 2 m2

The center of mass of an object is also the position at which the entire mass of the object could be concentrated, and still have the same "average motion" as the entire object does. The center of mass is vital to understanding the dynamics of a system of particles. For example, if we throw the "dumbell" shown in Figure 1, then the center of mass will follow a fairly simple path (roughly parabolic) whereas the ends will rotate around the center of mass, creating complicated paths as shown in Figure 2.

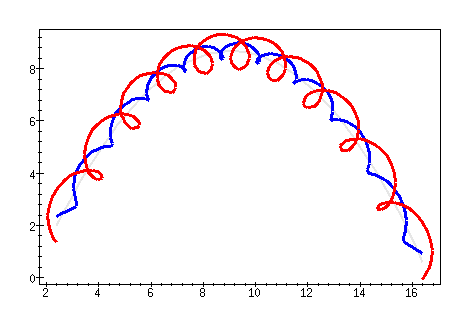
Figure 2: The motion of a rotating dumbell-shaped object.

Many Point-Masses

Suppose a rigid body is composed of n particles connected in a straight line. If the i th particle is located at x_i and has mass m_i , then the location of the center of mass for the system is x_cm where

(m1 + m2 + . . . + m_n)x_cm = m1 x1 + . . . + m_n x_n

The derivation of this equation involves finding the "pivot point" where the torques induced by the weight of each particle cancel out.

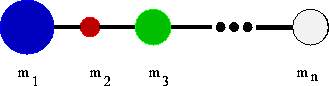
Figure 3: A rigid collection of n particles.

Note that this equation indicates why the rigid body "acts like" a point mass located at the center of mass. If we imagine trying to balance the whole system on a pivot located at the origin, each individual mass exerts a torque of m_i x_i . The net torque at the origin is then just the sum of the individual torques, which is the right hand side of the equation above. On the other hand, the torque exerted by a single particle of mass m_1 + ... + m_n located at x_cm is given by the left hand side of the equation.

If the particles are positioned in the plane at coordinates (x1,y1), ..., (x_n, y_n) , then the coordinates of the center of mass are defined by (x_cm , y_cm ) where

(m1 + . . . + m_n) x_cm = m1 x1 + . . . + m_n x_n

(m1 + . . . + m_n) y_cm = m1 y1 + . . . + m_n y_n

Question 1

When the shape of an object is symmetric, it is clear where the center of mass is. We can often use this observation to find the centers of mass for composite objects by decomposing them into symmetric regions that we can then treat as point masses.

Answer the following questions by interacting with the Exploring Centers of Mass page.

Experimentally find the center of mass for the "C-beam" cross-section (also called a "channel section" by engineers) on the next page. You'll know you are essentially correct when the background color turns yellow.
Decompose the C-beam into a union of rectangles by selecting one of the "Simple Subdivisions" from the menu at the bottom of the Exploration page, and find the center of mass for this subdivision. (Notice the black dots at the center of mass for each rectangle.) Does the center of mass of the C-beam change as the subdivision changes? Why does this make sense?
If we can decompose a composite region into simple symmetric regions, then the center of mass of the composite region may be found by replacing each simple region by a corresponding point-mass.
- Give a geometric argument for why the statement should be true. What should be the location of each point-mass? What should be the mass of each point-mass?
- Verify the statement above using the C-beam with "Simple Subdivision 1" as a concrete example. In particular, assume that the "legs" of the C-beam have width 0.62 and height 2.17, and the "roof" has width 4.34 and height 0.62. Assume the C-beam has constant density of 1. Determine both the location and mass of the three point-masses inside the "Simple Subdivision 1" on the Exploration page.
As this example shows, the center of mass of an object does not need to be on the object, yet it still makes sense to say that the center of mass is the location where the center of mass would balance on your finger and be perfectly level. Explain how a physical model of the C-beam can be made to balance at its center of mass. (Hint: consider line segments that connect the center of mass of the C-beam to the center of mass of each rectangle in the C-beam's decomposition.)

Next: Centers of Mass for Continuous Objects
Return to: Outline

The Geometry Center Calculus Development Team