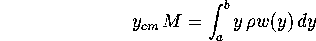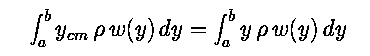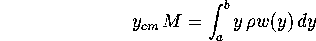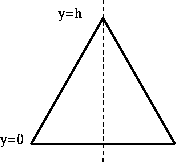Centers of Mass for Continuous Objects

For objects that are not symmetrical, we can find the center of mass by using the techniques of integral calculus. We cut the object into many small pieces and treat each of them as a point mass. The center of mass of the resulting system is approximately the same as the center of mass of the continuous object. As the number of pieces grows and their sizes shrink, the limit of the approximate solution approaches the true center of mass.

In practical terms, the summations turn into integrals. We want to think of the object as being cut into thin strips of small mass. For example, in Figure 4, the object is horizontally symmetric, so x_cm = 0 .

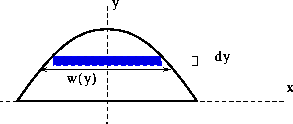
Figure 4: Slicing an irregular object into strips.

To find y_cm , we can chop the object into strips of small mass: dm = p w(y) dy , where p is the density of the object. Then y_cm satisfies the equation

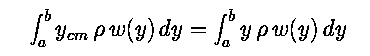
where a is the minimum value of y and b is the maximum value of y .

Observe that this formula doesn't seem to involve the mass of the object, only its shape. However, since y_cm is a constant, we can integrate the left hand side of the equation. Since the integral of the density over the region is the mass of the object, we have

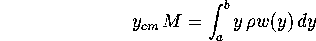
where M is the total mass of the object.

For this lab, we will always assume that our objects have uniform density. To make it clear that we are making this assumption, we call the point (x_cm, y_cm) defined by the above integral formula the centroid instead of the center of mass. Another way to think about it is that the centroid would be the center of mass if the object were cut out of a mathematically perfect, uniform material.

Question 2

Find the centroid of an isosceles triangle with height h and base length B .
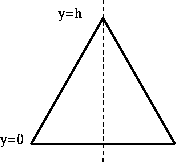
A trapezoid of height h has a bottom edge of length 2B and a top edge of length B and is symmetric with respect to the y -axis. Use the answer to the previous problem and your experience with Question 1 to compute the centroid for this trapezoid.

Next: Moments of Inertia
Return to: Outline

The Geometry Center Calculus Development Team