
![[More]](pix/Link.gif) ,
but was not able to produce a tight version with only one handle.
,
but was not able to produce a tight version with only one handle.
The core of the tight polyhedral model presented here
![[More]](pix/Link.gif) follows Kuiper's original drawings quite closely. Starting with this
initial projective plane, the top and bottom are cut off and a tube
added around the outside of the object to complete the tight immersion
of the projective plane with one handle.
follows Kuiper's original drawings quite closely. Starting with this
initial projective plane, the top and bottom are cut off and a tube
added around the outside of the object to complete the tight immersion
of the projective plane with one handle.
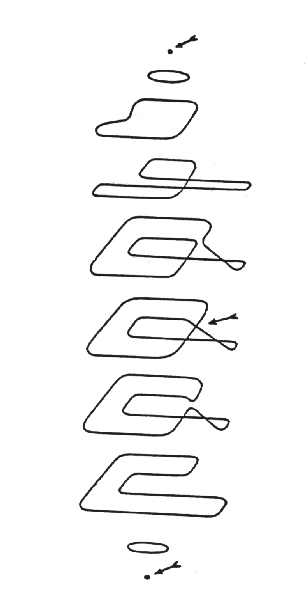
The following pages describe the relationship between Kuiper's drawings and the polyhedral level sets in more detail:
![[Right]](pix/Right.gif) Level 1: The initial circle.
Level 1: The initial circle.
![[Right]](pix/Right.gif) Level 2: Pulling one side across.
Level 2: Pulling one side across.
![[Right]](pix/Right.gif) Level 3: The initial self-intersection.
Level 3: The initial self-intersection.
![[Right]](pix/Right.gif) Level 4: Adding the triple point.
Level 4: Adding the triple point.
![[Right]](pix/Right.gif) Level 5: The critical level.
Level 5: The critical level.
![[Right]](pix/Right.gif) Level 6: After the critical level.
Level 6: After the critical level.
![[Right]](pix/Right.gif) Level 7: No more intersection.
Level 7: No more intersection.
![[Right]](pix/Right.gif) Level 8: The final circle.
Level 8: The final circle.
![[Left]](pix/Left.gif) Other pictures
Other pictures
![[Up]](pix/Up.gif) The polyhedral solution
The polyhedral solution
8/10/94 dpvc@geom.umn.edu --
The Geometry Center