The Polyhedral Solution:

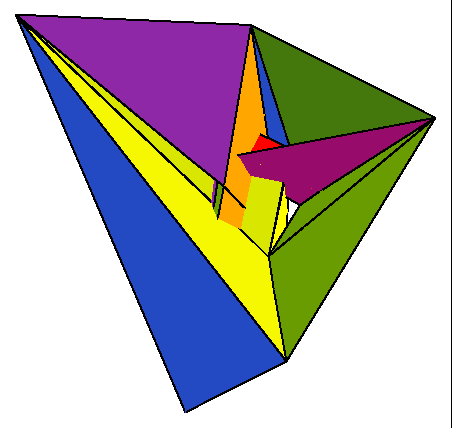
        Vertices:                       Faces:

       a = (-2,0,0)               abk bgk bgj bij gfj
       b = (0,0,0)                adl akl cdl flm clm
       c = (1,0,0)                hij ehi bci cim fim
       d = (0,1,0)                efi hkl hjl fjl ghk
       e = (-2,-1,2)
       f = (1,-1,2)               abe bef bcf cfg
       g = (1,1,2)                cgh cdh adh aeh
       h = (0,3,2)
       i = (-3/8,0,1/2)
       j = (1/2,1/4,1)
       k = (-1/4,7/12,7/6)
       l = (0,3/4,7/6)
       m = (1/4,0,1/2)

To see that this is really the projective plane with one handle, we compute the Euler characteristic: since there are 13 vertices, 42 edges, and 28 faces, the Euler characteristic is V - E + F = -1, as desired. Another way to see this is from the triangulation shown below, which shows the real projective plane (left) with two disks removed (grey) together with the tube that connects the two holes (right). The dotted line represents the self-intersection.

To see that it is an immersion, we need to check that the star of each vertex is embedded . This can be seen in the triangulation above, since the double locus does not contain any vertex. As expected, its image is the wedge of three circles, their common point forming the required triple point in the immersion .

To check that it is tight, one must show that all the edges of the convex hull are contained in the surface itself, and that any vertex that is not a vertex of the convex hull of the surface lies inside the convex hull of its neighbors .

To check these conditions, note that the convex hull of the surface is formed by 7 vertices (a, c, d, e, f, g, h), and the last 8 faces listed above contain all the edges of the convex hull. Of the remaining 6 vertices, two lie on the straight line segment between two neighbors (b lies on the segment ac, k lies on the segment ag), two lie within a triangle formed by three neighbors (i lies within triangle beh, and j within bfg), and two lie inside tetrahedra formed by four neighbors (l lies within acfh, and m lies within cfil).

The level sets for the height function in the direction of the z-axis closely resemble those originally given by Kuiper in [K2], where he describes a tight real projective plane with two handles.

The situation for smooth versions of the projective plane with a handle is markedly different; in fact, no tight immersion is possible in the smooth case . This polyhedral example is significant in that it represents one of only a handful of low-dimensional examples where the smooth and polyhedral theories differ in a significant way. The circumstances that provide for the difference in this specific case still deserve investigation, and should be a source of insight into both areas of study.