Conclusions

The computation of the envelope showed that indeed, the nephroid curve is part of the envelope of the family of reflected light rays, which explains how it ended up on the bottom of your coffee cup. But it also raises some questions:

Why are there extra factors x² y² present in the envelope? What curves are defined by the equations x² = 0 and y² = 0, and why should these curves be part of the envelope?
Why do these curves appear "doubled", i.e. why is it x² = 0 and y² = 0 rather than just x=0 and y=0?

The first two questions start to make sense when we look closely at the graph surface F(t,x,y)=0 shown below, and think about how its singularities relate to the envelope. You can click on the picture to generate an MPEG movie (646 Kbytes).

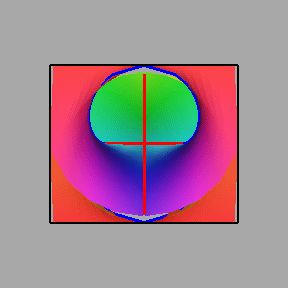
If you haven't read about projections, profiles, and envelopes, take a look at it now. Given an algebraic surface like F(t,x,y)=0, there is also a notion of its singular set which you can also read about.

Question 8

After reading about singular sets and profiles and envelopes, explain why the following statement is true: For any surface F(t,x,y)=0, the projection of its singular set onto the xy-plane will always show up as a subset of the t-profile of the surface.
Go back to the page about singular sets and look at the Maple computation of singularities on our graph surface. Which of the curves in the singular set project down to the "extra" pieces of the envelope x²=0 and y²=0?
Does the picture of the singular set inside the surface give you any clue as to why these curves appear "doubled", i.e. x²=0 and y²=0 rather than x=0 and y=0?

Previous: Computing the Envelope of a Family of Curves
Up: Introduction

Vic Reiner <reiner@math.umn.edu>

Frederick J. Wicklin <fjw@geom.umn.edu>

Last modified: Mon Apr 15 13:53:47 1996