Polyhedral vs Smooth Surfaces:

First, in the smooth case, the fold curves of almost all projections form disjoint components, so it is possible to count the number of components accurately. In the polyhedral case, the analogue of the fold curves are formed by fold edges (ones where the two triangles sharing that edge both project onto the same side of the edge), but any number of fold edges may come together at a single vertex. Thus it is not always possible to determine a canonical way to divide the fold edges into fold curves, and the number of components may change with different divisions into curves.

In the polyhedral model presented here , the projection onto the xy-plane has either one or two components (depending on how the choice is made at vertex h where four fold edges meet), but not the three predicted by Haab for the smooth case.

Second, the idea of a cusp and of locally convex curves is harder to formulate in the polyhedral case. One might begin by identifying analogous polyhedral structures, such as those pictured below, but this becomes more complicated when more than two fold edges occur at one vertex.

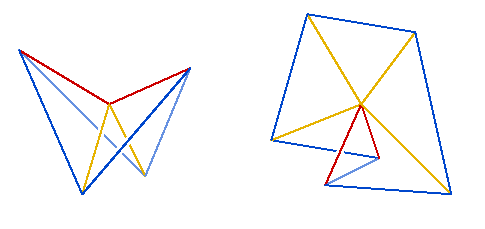
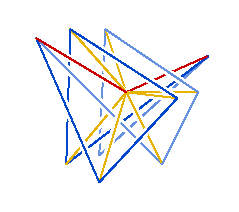
The problem is compounded by the fact that a star can wind around a vertex an arbitrary number of times with no visible effect on the angle between the fold edges, as shown in the rather complicated fold below. This makes computing the degree of a fold curve more complicated than in the smooth case (where small loops would be present to help out).