Abstract and Contents

List of all figures

Figure 1	Possible dynamics on two-dimensional stable and unstable manifolds.
Figure 2	The influence of the dynamics when iterating points.
Figure 3	The linearized stable and unstable manifolds of a circle of saddle-type.
Figure 4	The algorithm GLOBALIZE in pseudo-code.
Figure 5	The procedure ADDCIRCLE in pseudo-code.
Figure 6	Linear foliation near the invariant circle H.
Figure 7	The map acting on triangles of .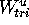
Figure 8	Initial mesh near a hyperbolic fixed point.
Figure 9	Mesh adaptation by adding extra leaves of the foliation.
Figure 10	Two different viewpoints of the stable manifold of the 3D-fattened Arnol'd family.
Figure 11	The unstable manifold of the Quasiperiodically forced Hénon map.
Figure 12	Heteroclinic tangle of two-dimensional stable and unstable manifolds of the Quasiperiodically forced Hénon map.
Figure 13	The unstable manifold of the origin of the example of a hyperbolic fixed point.
Figure 14	Comparing the condition of the mesh when leaves are added and when not.
Figure 15	The stable manifold of the Lorenz system computed by [Worfolk 1997].
Figure 16	The stable manifold of the Lorenz system computed with our algorith
Figure 17	Stable and unstable manifolds of the normal form of the Hopf-Hopf bifurcation.
Figure 18	A situation where the Foliation Condition is not satisfied.

Abstract and Contents

Written by: Bernd Krauskopf & Hinke Osinga
Created: May 27 1997 --- Last modified: Tue Jun 10 13:22:16 1997